In the previous section we saw some solved examples which illustrates the application of 'scales'. In this section, we will see some more examples. Later in this section we will see a comparison between Drawings and Models. We will also discuss about Reducing scales and Enlarging scales.
Solved example 3.11
The fig.3.11 below shows the drawing of two rooms. The smaller room is within the larger room.
The draughtsman forgot to write the length and width of the smaller room. Also he forgot to write the scale of the drawing. Various segments in the drawing were measured, and the following data was obtained:
ab = 10 cm, ad = 8 cm, ef = 4 cm, fg = 3 cm.
With this data, and the details already shown in the drawing, can you calculate those three missing information?
Solution:
Let us denote the actual measurements by Capital letters, and the corresponding lengths in the drawing by Small letters. So we can write:
• AB = 500 cm (written in drawing); ab = 10 cm (measured from drawing)
• AD = 400 cm (written in drawing); ad = 8 cm (measured from drawing)
• EF =?; ef = 4 cm (measured from drawing)
• FG =?; fg = 3 cm (measured from drawing)
• Let the scale be 1:S. Then a segment with an actual length of 'S' cm will have a length of 1 cm in the drawing.
The fig.3.11 below shows the drawing of two rooms. The smaller room is within the larger room.
 |
| Fig.3.11 |
The draughtsman forgot to write the length and width of the smaller room. Also he forgot to write the scale of the drawing. Various segments in the drawing were measured, and the following data was obtained:
ab = 10 cm, ad = 8 cm, ef = 4 cm, fg = 3 cm.
With this data, and the details already shown in the drawing, can you calculate those three missing information?
Solution:
Let us denote the actual measurements by Capital letters, and the corresponding lengths in the drawing by Small letters. So we can write:
• AB = 500 cm (written in drawing); ab = 10 cm (measured from drawing)
• AD = 400 cm (written in drawing); ad = 8 cm (measured from drawing)
• EF =?; ef = 4 cm (measured from drawing)
• FG =?; fg = 3 cm (measured from drawing)
• Let the scale be 1:S. Then a segment with an actual length of 'S' cm will have a length of 1 cm in the drawing.
Columns (v), (vi) and (vii) should give the same ratio 0.02. So we can write:
(i) 4/EF = 0.02 ⇒ EF = 4/0.02 = 200 cm
(ii) 3/FG = 0.02 ⇒ FG = 3/0.02 = 150 cm
(iii) 1/S = 0.02 ⇒ S = 1/0.02 = 50 cm
Thus we get all the missing information. The scale of the map is 1 cm = 50 cm ⇒ 1:50
In the above problem, the drawing is incomplete. Such a drawing should not be used for practical purposes. It is not allowed to measure lengths from drawings. If we come across such a drawing, all proceedings should be immediately stopped, and proper authorities must be informed.
However, such an incomplete drawing helps us to test our skills. An old drawing or map may lose many essential details. We can use our skills to obtain possible information from them. But our findings should not be put to use with out permission from senior authorities.
Drawing vs Model.
The drawings that we saw so far are two dimensional. They are drawn on a sheet of paper, and they give us two dimensions: Length and Width. But a Model is three dimensional. It gives Length, Width and Thickness. It can be made with materials like wood, cardboard, plaster of paris, fibreboard etc., Some images of models can be seen here. Just like in a drawing, in a model also, the actual dimensions are reduced proportionately, using an appropriate scale. We will now see a solved example related to models.
Solved example 3.12
The actual width of a car is 1.6 m. The width of it's model is 32 cm.
(i) What is the length of the model, if the actual length of the car is 4.8 m
(ii) What is the actual height of the car, if the height of the model is 36 cm ?
(iii) What is the scale of the model?
Solution:
The table is shown below:
• The actual width is given as 1.6 m, and the width of the model is given as 32 cm. This information is used to fill up column (iii).
• The length of the model is denoted as l. This l and 4.8 m should give the same ratio 20.00.
• The actual height is denoted as H. 36 cm and H should give the same ratio 20.00
• Let the scale be 1:S. Then a segment with an actual length of 'S' m will have a length of 1 cm in the model.
So we can write:
(i) l/4.8 = 20.00 ⇒ l = 4.8 × 20 = 96 cm
(ii) 36/H = 20.00 ⇒ H = 36/20 = 1.8 m
(iii) 1/S = 20.00 ⇒ S = 1/20 = 0.05
So we got all the required answers. The scale is 1 cm = 0.05 m. To write this using the ':' symbol, we need same unit on both sides.
We have 1 m = 100 cm. So 0.05 m = 5 cm. Thus the scale is 1:5
Solved example 3.13
A room is square in shape. It is drawn to a scale of 1:50. The length of the sides of the square on the drawing is 7 cm.
(i) What is the actual length of the side?
(ii) If the length of the side on the drawing is to be 14 cm, at what scale should it be drawn?
Solution:
(i) The scale is given as 1:50. So, if there is a segment with actual length 50 cm, it will be shown as 1 cm in the drawing. This information fills up the first column of the table. The length on the drawing l1 is given as 7 cm. So this 7 cm, and it’s corresponding actual length L1 fills another column. Thus the table can be formed as:
7 and L1 gives the same constant. So we can write:
7/L1 = 0.02 ⇒ L1 = 7/0.02 = 350 cm
(ii) In the second part, they are asking the value of a new scale. That means a entirely different new drawing. So we have to make an entirely different new table. See details here. For filling up this new table, we get one important information from part (i). That is., the actual length of the side of the square room. We obtained it as 350 cm. In the new drawing, this 350 cm has to become 14 cm. So 350 and 14 fills up the first column. To fill up the second column, let us put the scale as 1:S. Any segment having an actual length S will become 1 cm in the drawing. So 1 and S fills up the second column. This is shown below:
1 and S should give the same ratio of 0.04. So we can write:
1/S = 0.04 ⇒ S = 1/0.04 = 25. So the required scale is 1:25
So we got all the required answers. The scale is 1 cm = 0.05 m. To write this using the ':' symbol, we need same unit on both sides.
We have 1 m = 100 cm. So 0.05 m = 5 cm. Thus the scale is 1:5
Solved example 3.13
A room is square in shape. It is drawn to a scale of 1:50. The length of the sides of the square on the drawing is 7 cm.
(i) What is the actual length of the side?
(ii) If the length of the side on the drawing is to be 14 cm, at what scale should it be drawn?
Solution:
(i) The scale is given as 1:50. So, if there is a segment with actual length 50 cm, it will be shown as 1 cm in the drawing. This information fills up the first column of the table. The length on the drawing l1 is given as 7 cm. So this 7 cm, and it’s corresponding actual length L1 fills another column. Thus the table can be formed as:
7 and L1 gives the same constant. So we can write:
7/L1 = 0.02 ⇒ L1 = 7/0.02 = 350 cm
(ii) In the second part, they are asking the value of a new scale. That means a entirely different new drawing. So we have to make an entirely different new table. See details here. For filling up this new table, we get one important information from part (i). That is., the actual length of the side of the square room. We obtained it as 350 cm. In the new drawing, this 350 cm has to become 14 cm. So 350 and 14 fills up the first column. To fill up the second column, let us put the scale as 1:S. Any segment having an actual length S will become 1 cm in the drawing. So 1 and S fills up the second column. This is shown below:
1 and S should give the same ratio of 0.04. So we can write:
1/S = 0.04 ⇒ S = 1/0.04 = 25. So the required scale is 1:25
Reducing Scale and Enlarging Scale
The scales that we have seen so far in this chapter, were used to reduce the size of large objects so that, they will fit into standard sizes of paper. They are called Reducing Scales. Some times we will have to prepare drawings of small objects. For example, parts of a wrist watch, Parts of electric appliances, diagrams of micro organisms etc., Obviously, we cannot draw them to actual size. We will not even be able to draw them properly. In such cases, we use Enlarging Scales.
Fig.3.4 that we saw earlier showed us how each 100 cm in the real object shrinks to 1 cm in the drawing. Similarly, fig.3.6 showed us how each 50 cm in the real object shrinks to 1 cm in the drawing. Those where for large objects. But for small objects, we want the opposite of 'shrink'. That is., we want 'expansion'. We want each 1 cm (there will only be a very few 'one centimetres' in a small object) in the real object to expand to a higher value.
We will now see some solved examples based on Enlarging scales.
Solved example 3.14
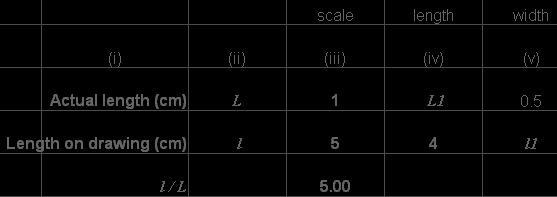
A small component of an electronic device is rectangular in shape. It is drawn at an enlarging scale of 5:1. In the drawing, the length is 4 cm.
(i) What is the actual length?
(ii) If the actual width is 0.5 cm, what is the width in the drawing?
Solution:
The scale is given as 5:1. So if any segment has an actual length of 1 cm, it will have a length of 5 cm in the drawing. We can use this 5 and 1 to fill up the first column. Also we have:
• Actual length L1 has to be calculated. Length on drawing l1 is given as 4 cm. These two fill up the second column
• Actual width L2 is given as 0.5 cm. Width on drawing l2 has to be calculated. These two fill up the third column.
Thus the table is formed as:
(i) 4 and L1 should give the same ratio 5.00. So we can write:
4/L1 = 5.00 ⇒ L1 = 4/5.00 = 0.8 cm
(ii) l1 and 0.5 should give the same ratio 5.00. So we can write:
l1/0.5 = 5.00 ⇒ l1 = 0.5 × 5 = 2.5 cm
Solved example 3.15
A small component of a wrist watch is square in shape. It is drawn to a scale of 10:1. On the drawing, the square has a side of 5 cm.
(i) What is the actual length of the side of the square?
(ii) What scale should we use, if the square should have a side of 15 cm on the drawing?
Solution:
(i) The scale is given as 10:1. So, if there is a segment with actual length 1 cm, it will be shown as 10 cm in the drawing. This information fills up the first column of the table. The length on the drawing l1 is given as 5 cm. So this 5 cm, and it’s corresponding actual length L1 fills another column. Thus the table can be formed as:
5 and L1 gives the same constant. So we can write:
5/L1 = 10 ⇒ L1 = 5/10 = 0.5 cm. So the actual length of the side is 0.5 cm
(ii) In the second part, they are asking the value of a new scale. That means a entirely different new drawing. So we have to make an entirely different new table. See details here. For filling up this new table, we get one important information from part (i). That is., the actual length of the side of the square. We obtained it as 0.5 cm. In the new drawing, this 0.5 cm has to become 15 cm. So 0.5 and 15 fills up the first column. To fill up the second column, let us put the scale as S:1. Any segment having an actual length 1 will become S cm in the drawing. So 1 and S fills up the second column. This is shown below:
1 and S should give the same ratio of 30.00. So we can write:
S/1 = 30.00 ⇒ S = 1×30 = 30. So the required scale is 30:1
We have completed the discussions on the 'Direct proportions and it's applications'. In the next section, we will see the 'Graphs of Direct proportions'.
Fig.3.4 that we saw earlier showed us how each 100 cm in the real object shrinks to 1 cm in the drawing. Similarly, fig.3.6 showed us how each 50 cm in the real object shrinks to 1 cm in the drawing. Those where for large objects. But for small objects, we want the opposite of 'shrink'. That is., we want 'expansion'. We want each 1 cm (there will only be a very few 'one centimetres' in a small object) in the real object to expand to a higher value.
Let us assume that 1 cm expands to 5 cm. If there is a 3 cm long object, then each 1 cm will expand to 5 cm. So the total length in the drawing will be 15 cm. This is shown in the fig.3.12 below:
 |
| Fig.3.12 |
If it is assumed that 1 cm expands to 2 cm, then each one cm expands to 2 cm, and the length of the drawing will be 6 cm. This is shown in the fig below:
 |
| Fig.3.13 |
Now we will see how to indicate an enlarging scale in drawings. We have seen it for a reducing scale earlier in fig.3.8 The rules are the same: Left side is for measurements in the drawing, and right side is for measurements in the real object. So fig.3.8 can be modified as given below:
 |
| Fig.3.14 |
Note that, in a reducing scale, the value at the right side shrinks to the value at the left side. While in an enlarging scale, value at the right side expands to the value at the left side.
We will now see some solved examples based on Enlarging scales.
Solved example 3.14
A small component of an electronic device is rectangular in shape. It is drawn at an enlarging scale of 5:1. In the drawing, the length is 4 cm.
(i) What is the actual length?
(ii) If the actual width is 0.5 cm, what is the width in the drawing?
Solution:
The scale is given as 5:1. So if any segment has an actual length of 1 cm, it will have a length of 5 cm in the drawing. We can use this 5 and 1 to fill up the first column. Also we have:
• Actual length L1 has to be calculated. Length on drawing l1 is given as 4 cm. These two fill up the second column
• Actual width L2 is given as 0.5 cm. Width on drawing l2 has to be calculated. These two fill up the third column.
Thus the table is formed as:
(i) 4 and L1 should give the same ratio 5.00. So we can write:
4/L1 = 5.00 ⇒ L1 = 4/5.00 = 0.8 cm
(ii) l1 and 0.5 should give the same ratio 5.00. So we can write:
l1/0.5 = 5.00 ⇒ l1 = 0.5 × 5 = 2.5 cm
Solved example 3.15
A small component of a wrist watch is square in shape. It is drawn to a scale of 10:1. On the drawing, the square has a side of 5 cm.
(i) What is the actual length of the side of the square?
(ii) What scale should we use, if the square should have a side of 15 cm on the drawing?
Solution:
(i) The scale is given as 10:1. So, if there is a segment with actual length 1 cm, it will be shown as 10 cm in the drawing. This information fills up the first column of the table. The length on the drawing l1 is given as 5 cm. So this 5 cm, and it’s corresponding actual length L1 fills another column. Thus the table can be formed as:
5 and L1 gives the same constant. So we can write:
5/L1 = 10 ⇒ L1 = 5/10 = 0.5 cm. So the actual length of the side is 0.5 cm
(ii) In the second part, they are asking the value of a new scale. That means a entirely different new drawing. So we have to make an entirely different new table. See details here. For filling up this new table, we get one important information from part (i). That is., the actual length of the side of the square. We obtained it as 0.5 cm. In the new drawing, this 0.5 cm has to become 15 cm. So 0.5 and 15 fills up the first column. To fill up the second column, let us put the scale as S:1. Any segment having an actual length 1 will become S cm in the drawing. So 1 and S fills up the second column. This is shown below:
1 and S should give the same ratio of 30.00. So we can write:
S/1 = 30.00 ⇒ S = 1×30 = 30. So the required scale is 30:1
We have completed the discussions on the 'Direct proportions and it's applications'. In the next section, we will see the 'Graphs of Direct proportions'.






No comments:
Post a Comment