In the previous section we learned about some properties of equivalent fractions. In this section we will see two more properties.
Consider the following patterns:
■ 1/3 and 2/6 are two equivalent fractions. Some changes are made to them. The changed form is written on the right side of the arrow. What changes are made?
• The numerator of the second fraction became denominator of the first fraction. Thus 1/3 became 1/2
• The denominator of the first fraction became numerator of the second fraction. Thus 2/6 became 3/6
• In short, numerator and denominator were interchanged. Note that the interchange occurred only in one direction as shown below:
• We have the 'result after interchange' on the right side of the arrow. What peculiarity do we see on the result?
• The peculiarity is: Result is also a pair of equivalent fractions.
■ 2/5 and 4/10 are two equivalent fractions. Some changes are made to them. The changed form is written on the right side of the arrow. What changes are made?
• The numerator of the second fraction became denominator of the first fraction. Thus 2/5 became 2/4
• The denominator of the first fraction became numerator of the second fraction. Thus 4/10 became 5/10
• In short, numerator and denominator were interchanged. Note that, as in the previous case, the interchange occurred only in one direction
• We have the 'result after interchange' on the right side of the arrow. What peculiarity do we see on the result?
• The peculiarity is: Result is also a pair of equivalent fractions.
■ 3/7 and 9/21 are two equivalent fractions. Some changes are made to them. The changed form is written on the right side of the arrow. What changes are made?
• The numerator of the second fraction became denominator of the first fraction. Thus 3/7 became 3/9
• The denominator of the first fraction became numerator of the second fraction. Thus 9/21 became 7/21
• In short, numerator and denominator were interchanged. Note that, as in the previous case, the interchange occurred only in one direction
• We have the 'result after interchange' on the right side of the arrow. What peculiarity do we see on the result?
• The peculiarity is: Result is also a pair of equivalent fractions.
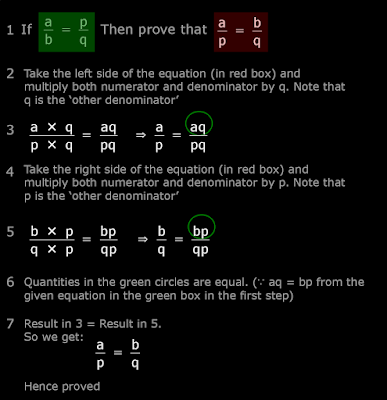
This is also a very useful property of equivalent fractions, which finds application in many areas of science and engineering. We need to prove the property algebraically:
Consider another pattern:
■ In the first pattern, we have two equivalent fractions: 3/4 and 9/12
• Based on them, a new fraction is formed
• The numerator of the new fraction consists of the sum of two products:
♦ First product is (m × first numerator). In this case m = 2
♦ Second product is (n × second numerator). In this case n = 5
• The denominator of the new fraction consists of the sum of two products:
♦ First product is (m × first denominator). m is same as above
♦ Second product is (n × second denominator). n is same as above
• Thus we get the new fraction. In this case it is 51/68
• This new fraction is equal to the first of the two original equivalent fractions. (dividing both numerator and denominator by 17, we get 3/4)
■ In the second pattern, we have two equivalent fractions: 4/7 and 8/14
• Based on them, a new fraction is formed
• The numerator of the new fraction consists of the sum of two products:
♦ First product is (m × first numerator). In this case m = 9
♦ Second product is (n × second numerator). In this case n = 2
• The denominator of the new fraction consists of the sum of two products:
♦ First product is (m × first denominator). m is same as above
♦ Second product is (n × second denominator). n is same as above
• Thus we get the new fraction. In this case it is 52/91
• This new fraction is equal to the first of the two original equivalent fractions. (dividing both numerator and denominator by 17, we get 1/2)
■ In the third pattern, we have two equivalent fractions: 1/2 and 2/4
• Based on them, a new fraction is formed
• The numerator of the new fraction consists of the sum of two products:
♦ First product is (m × first numerator). In this case m = 3
♦ Second product is (n × second numerator). In this case n = 7
• The denominator of the new fraction consists of the sum of two products:
♦ First product is (m × first denominator). m is same as above
♦ Second product is (n × second denominator). n is same as above
• Thus we get the new fraction. In this case it is 17/34
• This new fraction is equal to the first of the two original equivalent fractions. (dividing both numerator and denominator by 13, we get 4/7)
This property is also a very important. We need to prove the property algebraically:
We have learnt the important properties of equivalent fractions. We will now see some solved examples.
Solved example 5.34
• The numerator of a fraction is: Square of a number + 1
• The denominator of the fraction is: Square of the number – 1
• If the value of the fraction is 221/220, which is the number?
Solution:
• Let the number be 'k'
• Square of the number + 1 = k2 + 1
• Square of the number -1 = k2 – 1
So the calculation steps can be written as follows:
In this problem, a = k2 + 1 , b = k2 – 1, p = 221 and q = 220
Another method:
We can also use cross multiplication to solve this problem:
Solved example 5.35
• The numerator of a fraction is: Square of a number + the number
• The denominator of the fraction is: Square of the number – the number
• If the value of the fraction is 11⁄2 , which is the number?
Solution:
• Let the number be 'r'
• Square of the number + the number = r2 + r
• Square of the number -the number = r2 - r
• So we can write:
In this problem, a = r2 + r , b = r2 – r, p = 3 and q = 2
Another method:
We can also use cross multiplication to solve this problem:
So we have seen the properties of equivalent fractions. In the next section we will see properties of unequal fractions.
Consider the following patterns:
■ 1/3 and 2/6 are two equivalent fractions. Some changes are made to them. The changed form is written on the right side of the arrow. What changes are made?
• The numerator of the second fraction became denominator of the first fraction. Thus 1/3 became 1/2
• The denominator of the first fraction became numerator of the second fraction. Thus 2/6 became 3/6
• In short, numerator and denominator were interchanged. Note that the interchange occurred only in one direction as shown below:
• We have the 'result after interchange' on the right side of the arrow. What peculiarity do we see on the result?
• The peculiarity is: Result is also a pair of equivalent fractions.
■ 2/5 and 4/10 are two equivalent fractions. Some changes are made to them. The changed form is written on the right side of the arrow. What changes are made?
• The numerator of the second fraction became denominator of the first fraction. Thus 2/5 became 2/4
• The denominator of the first fraction became numerator of the second fraction. Thus 4/10 became 5/10
• In short, numerator and denominator were interchanged. Note that, as in the previous case, the interchange occurred only in one direction
• We have the 'result after interchange' on the right side of the arrow. What peculiarity do we see on the result?
• The peculiarity is: Result is also a pair of equivalent fractions.
■ 3/7 and 9/21 are two equivalent fractions. Some changes are made to them. The changed form is written on the right side of the arrow. What changes are made?
• The numerator of the second fraction became denominator of the first fraction. Thus 3/7 became 3/9
• The denominator of the first fraction became numerator of the second fraction. Thus 9/21 became 7/21
• In short, numerator and denominator were interchanged. Note that, as in the previous case, the interchange occurred only in one direction
• We have the 'result after interchange' on the right side of the arrow. What peculiarity do we see on the result?
• The peculiarity is: Result is also a pair of equivalent fractions.
This is also a very useful property of equivalent fractions, which finds application in many areas of science and engineering. We need to prove the property algebraically:
Consider another pattern:
• Based on them, a new fraction is formed
• The numerator of the new fraction consists of the sum of two products:
♦ First product is (m × first numerator). In this case m = 2
♦ Second product is (n × second numerator). In this case n = 5
• The denominator of the new fraction consists of the sum of two products:
♦ First product is (m × first denominator). m is same as above
♦ Second product is (n × second denominator). n is same as above
• Thus we get the new fraction. In this case it is 51/68
• This new fraction is equal to the first of the two original equivalent fractions. (dividing both numerator and denominator by 17, we get 3/4)
■ In the second pattern, we have two equivalent fractions: 4/7 and 8/14
• Based on them, a new fraction is formed
• The numerator of the new fraction consists of the sum of two products:
♦ First product is (m × first numerator). In this case m = 9
♦ Second product is (n × second numerator). In this case n = 2
• The denominator of the new fraction consists of the sum of two products:
♦ First product is (m × first denominator). m is same as above
♦ Second product is (n × second denominator). n is same as above
• Thus we get the new fraction. In this case it is 52/91
• This new fraction is equal to the first of the two original equivalent fractions. (dividing both numerator and denominator by 17, we get 1/2)
■ In the third pattern, we have two equivalent fractions: 1/2 and 2/4
• Based on them, a new fraction is formed
• The numerator of the new fraction consists of the sum of two products:
♦ First product is (m × first numerator). In this case m = 3
♦ Second product is (n × second numerator). In this case n = 7
• The denominator of the new fraction consists of the sum of two products:
♦ First product is (m × first denominator). m is same as above
♦ Second product is (n × second denominator). n is same as above
• Thus we get the new fraction. In this case it is 17/34
• This new fraction is equal to the first of the two original equivalent fractions. (dividing both numerator and denominator by 13, we get 4/7)
This property is also a very important. We need to prove the property algebraically:
We have learnt the important properties of equivalent fractions. We will now see some solved examples.
Solved example 5.34
• The numerator of a fraction is: Square of a number + 1
• The denominator of the fraction is: Square of the number – 1
• If the value of the fraction is 221/220, which is the number?
Solution:
• Let the number be 'k'
• Square of the number + 1 = k2 + 1
• Square of the number -1 = k2 – 1
So the calculation steps can be written as follows:
In this problem, a = k2 + 1 , b = k2 – 1, p = 221 and q = 220
Another method:
We can also use cross multiplication to solve this problem:
Solved example 5.35
• The numerator of a fraction is: Square of a number + the number
• The denominator of the fraction is: Square of the number – the number
• If the value of the fraction is 11⁄2 , which is the number?
Solution:
• Let the number be 'r'
• Square of the number + the number = r2 + r
• Square of the number -the number = r2 - r
• So we can write:
In this problem, a = r2 + r , b = r2 – r, p = 3 and q = 2
Another method:
We can also use cross multiplication to solve this problem:
So we have seen the properties of equivalent fractions. In the next section we will see properties of unequal fractions.











No comments:
Post a Comment